Are you asking for 'write a piecewise function for absolute value'? Here you can find the questions and answers on the subject.
Table of contents
- Write a piecewise function for absolute value in 2021
- How to write a piecewise function from a graph
- Write the absolute value function as a piecewise function calculator
- Piecewise functions worksheet
- Write a rule for an absolute value function
- Absolute value piecewise functions khan academy
- Absolute value and piecewise functions worksheet answers
- Example of a piecewise function
Write a piecewise function for absolute value in 2021
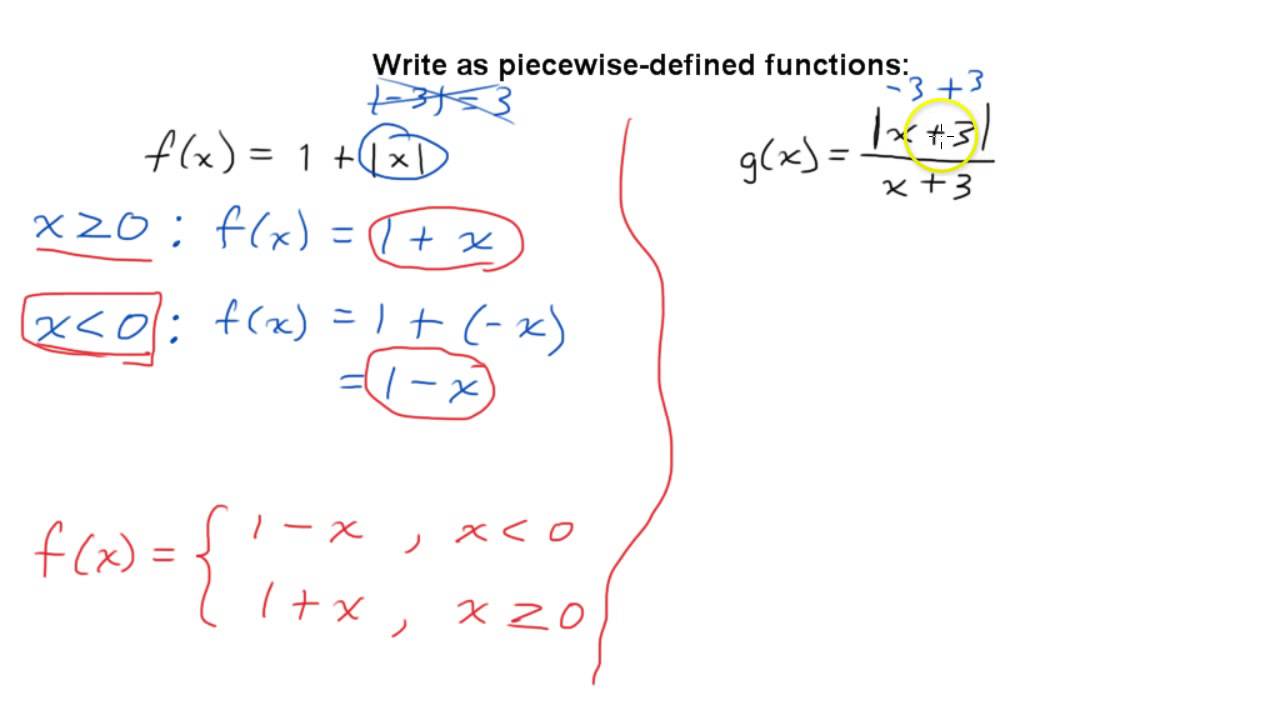 This image demonstrates write a piecewise function for absolute value.
This image demonstrates write a piecewise function for absolute value.
How to write a piecewise function from a graph
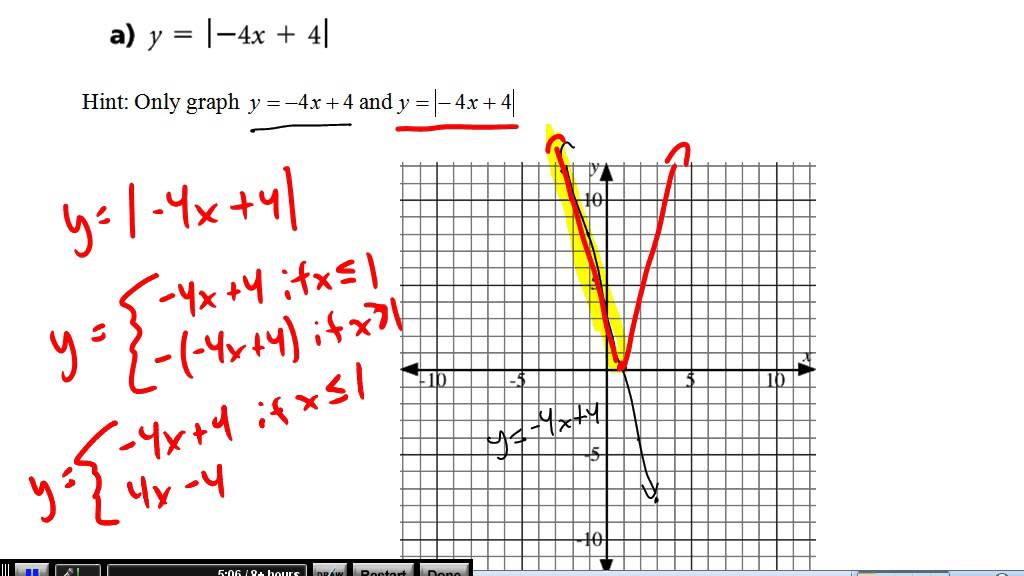 This picture demonstrates How to write a piecewise function from a graph.
This picture demonstrates How to write a piecewise function from a graph.
Write the absolute value function as a piecewise function calculator
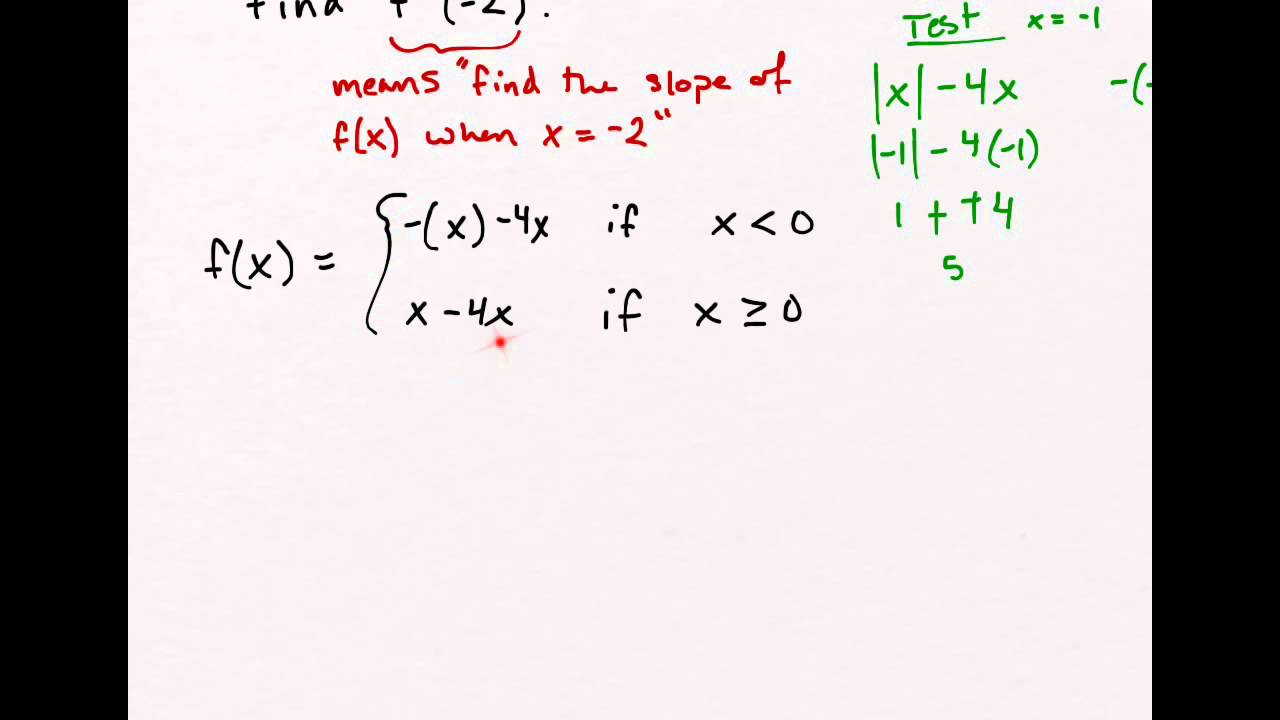 This image representes Write the absolute value function as a piecewise function calculator.
This image representes Write the absolute value function as a piecewise function calculator.
Piecewise functions worksheet
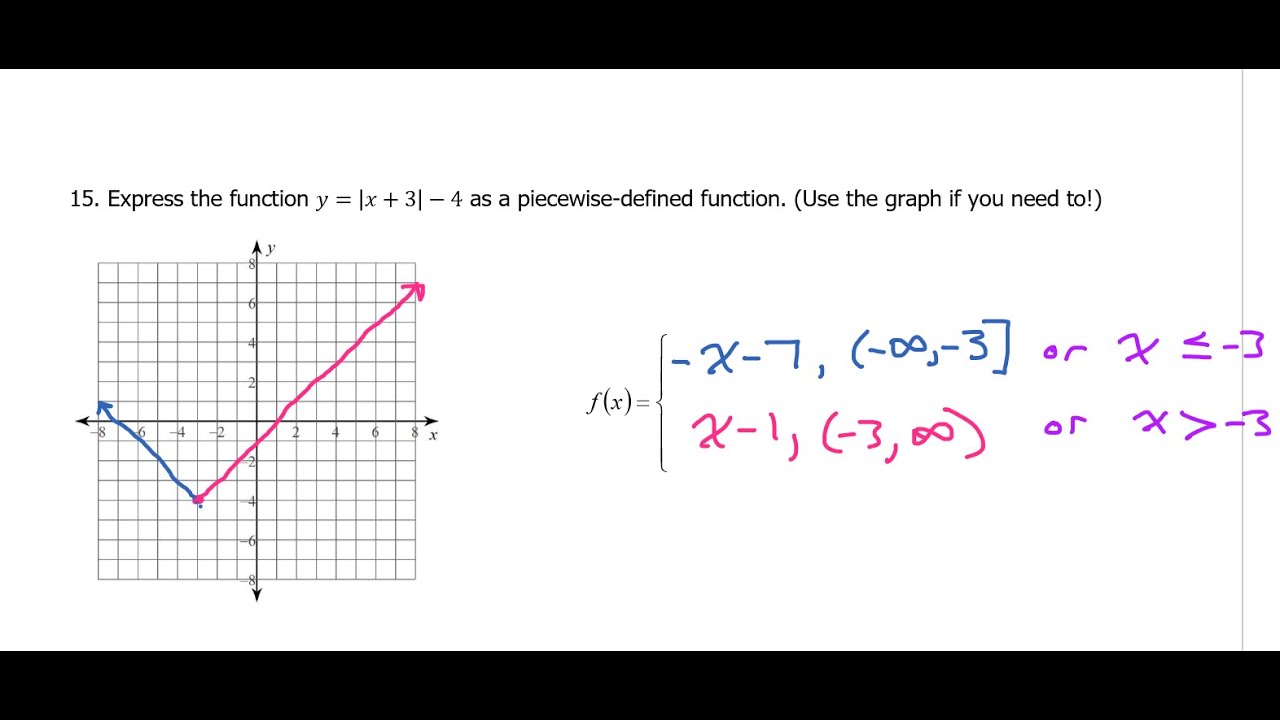 This image representes Piecewise functions worksheet.
This image representes Piecewise functions worksheet.
Write a rule for an absolute value function
 This picture shows Write a rule for an absolute value function.
This picture shows Write a rule for an absolute value function.
Absolute value piecewise functions khan academy
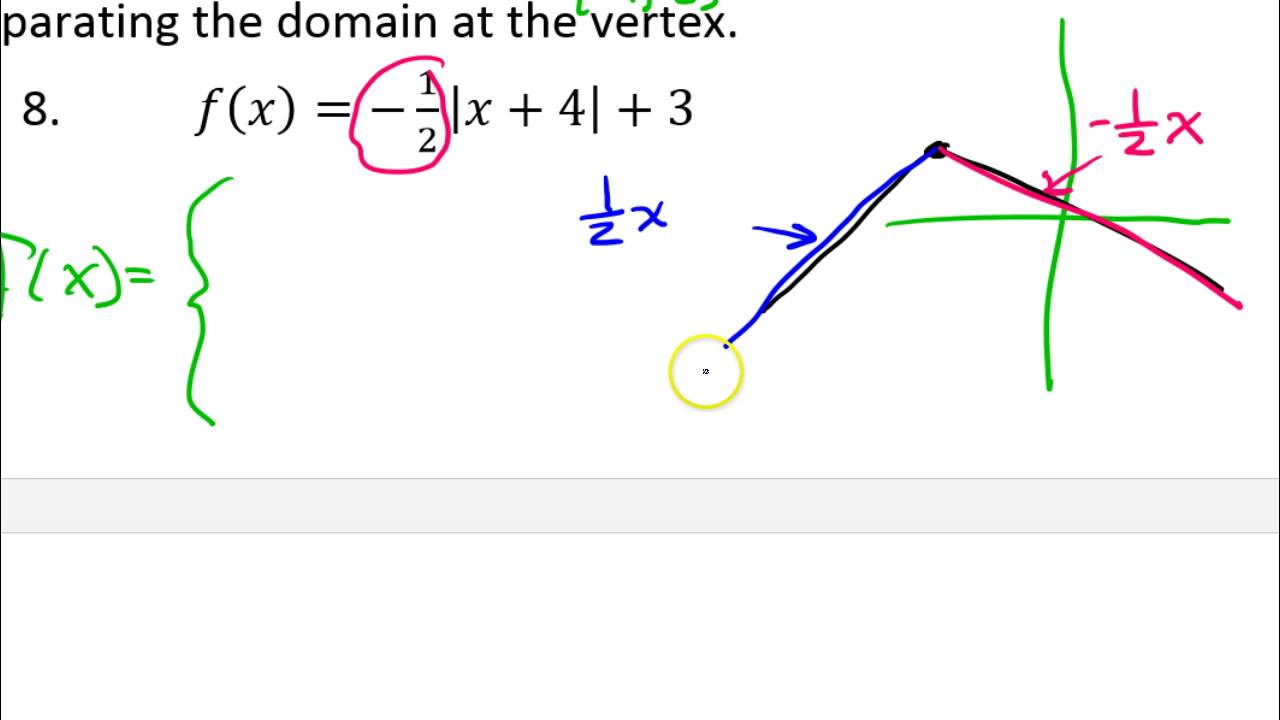 This image representes Absolute value piecewise functions khan academy.
This image representes Absolute value piecewise functions khan academy.
Absolute value and piecewise functions worksheet answers
 This image representes Absolute value and piecewise functions worksheet answers.
This image representes Absolute value and piecewise functions worksheet answers.
Example of a piecewise function
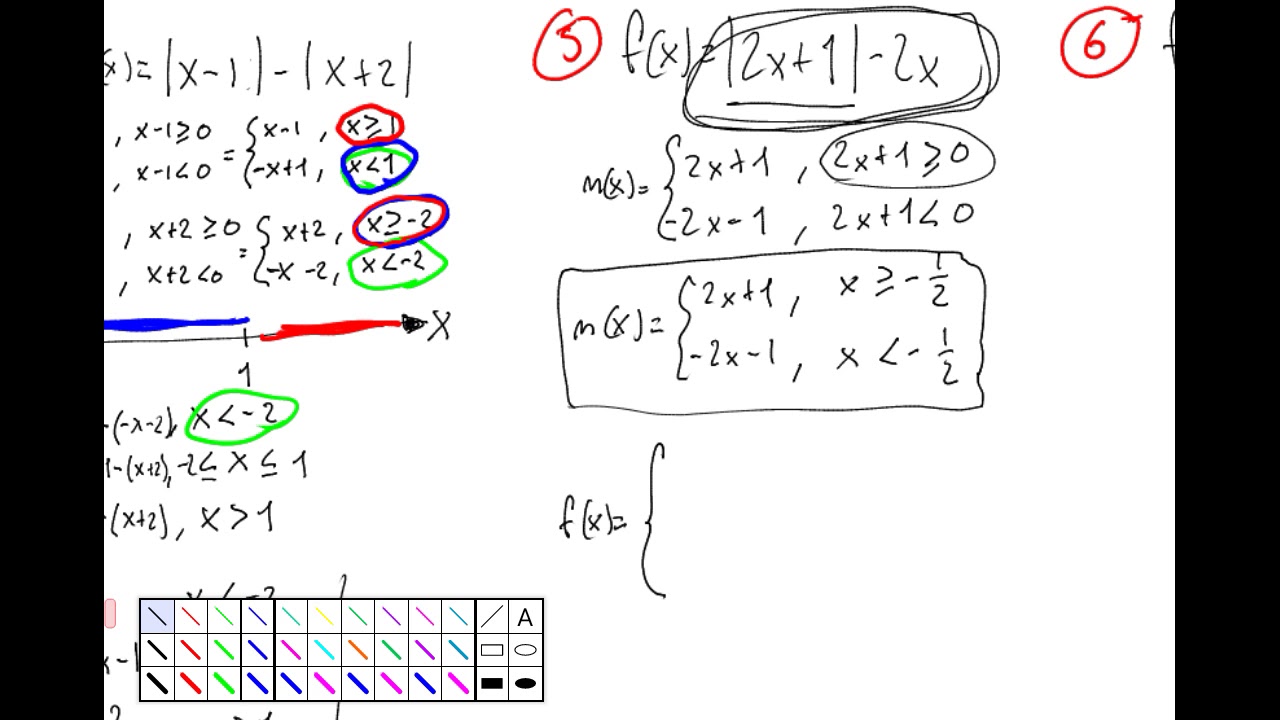 This picture illustrates Example of a piecewise function.
This picture illustrates Example of a piecewise function.
How to write absolute value as piecewise taisee Island?
First find the split. It occurs where the argument equals zero. x – 1 = 0 when x = 1. The split is at x=1. Determine where the graph is positive vs. negative (reflected). Substitute values into the argument to see if the outcome is positive or negative.
How to level up absolute value in Algebra?
Scale & reflect absolute value graphs Get 3 of 4 questions to level up! Graph absolute value functions Get 3 of 4 questions to level up! Evaluate piecewise functions Get 3 of 4 questions to level up! Evaluate step functions Get 3 of 4 questions to level up! Piecewise functions graphs Get 3 of 4 questions to level up!
How to express absolute value equations as piecewise functions?
For x < − 1, we have x + 1 < 0 and so | x + 1 | = − ( x + 1). Similarly, x − 2 < x + 1 < 0 and so | x − 2 | = − ( x − 2). So on the interval ( − ∞, − 1) the function can be simplified as f ( x) = − 3 ( x − 2) + ( x + 1) = − 2 x + 7.
How to write f ( x ) as piecewise function?
Write f(x) = |x – 1| + 2 as a piecewise function. First find the split. It occurs where the argument equals zero. x – 1 = 0 when x = 1. The split is at x=1. Determine where the graph is positive vs. negative (reflected). Substitute values into the argument to see if the outcome is positive or negative.
Last Update: Oct 2021
Leave a reply
Comments
Dealva
23.10.2021 06:08Indeed by now you've probably seen the graph of the ups of economic value of x At some point. Can ane use scatter plot of ground and prediction equations?
Layvonne
20.10.2021 09:56Piecewise functions are non considered a social occasion family on their own. Absolute value social function graph.
Everardo
21.10.2021 06:25Many functions have mere rules, like for every x, coming back x². Rewrite the equality without the arbitrary value as letter a piecewise function.